基于最短折减路径法的边坡安全系数研究

郑永来; 吴卓睿

doi:10.19509/j.cnki.dzkq.2022.0224

基于最短折减路径法的边坡安全系数研究

doi: 10.19509/j.cnki.dzkq.2022.0224

郑永来,
吴卓睿^,

同济大学土木工程学院, 上海 200092

基金项目:

水利部土石坝破坏机理与防控技术重点实验室开放基金项目 YK319002

详细信息

作者简介:
郑永来(1964-), 男, 教授, 主要从事海洋岩土工程及边坡稳定性研究。E-mail: zyll@tongji.edu.cn

通讯作者:
吴卓睿(1993-), 男, 现正读土木工程专业博士学位, 主要从事地下工程及边坡稳定性研究。E-mail: wuzr@tongji.edu.cn

中图分类号: P642.2
计量
- 文章访问数: 949
- PDF下载量: 87
- 被引次数: 0
出版历程
- 收稿日期: 2022-07-15

Estimation of slope safety factor based on trajectory reduction method

Zheng Yonglai,
Wu Zhuorui^,

College of Civil Engineering, Tongji University, Shanghai 200092, China

摘要

摘要:
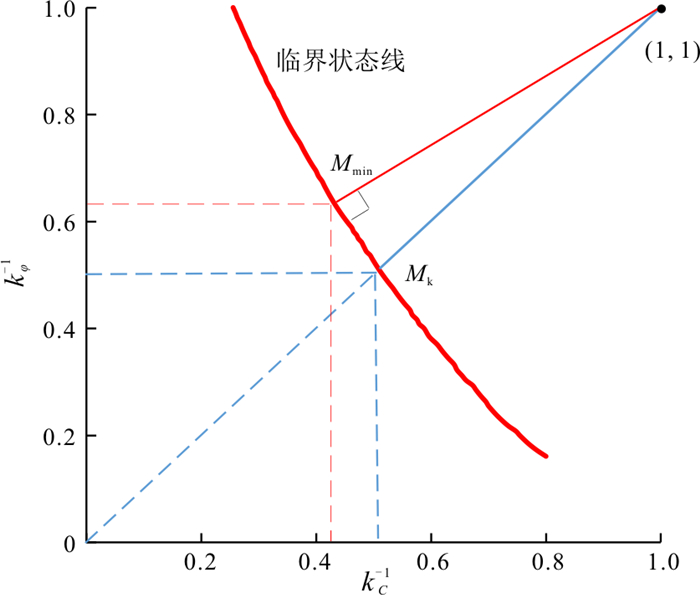
使用双参数折减方法分析边坡稳定性的研究较多, 如何把两个折减系数定义为单一的综合安全系数是目前研究的一项重要内容。Isakov提出的最短折减路径法能够保证在不同工况下得到最小安全系数, 但是该方法的缺点在于计算复杂, 不适合工程应用。通过有限元数值模拟, 利用最短折减路径方法计算不同强度黏土构成的不同坡度均质土坡的最小安全系数和对应的折减系数, 探索了最小安全系数与土的初始黏聚力、内摩擦角以及边坡坡度的关系, 分析了初始强度对折减系数的影响。结果表明, 相同坡度下不同强度的黏土边坡在失稳时, 最小安全系数对应的临界破坏强度相同。临界破坏强度与坡度近似成线性正相关关系。由此基于最短折减路径法提出了一种新的计算最小安全系数的方法, 该方法得到的安全系数与目前常用的极限平衡方法所得结果相近, 并且计算简单, 因此可以用于边坡稳定性分析。
- 边坡稳定分析 /
- 双参数折减 /
- 最短折减路径法 /
- 安全系数 /
- 临界破坏强度
Abstract:
Currently, double reduction method (DRM) is widely used in the field of slope stability. However, one of the main challenges of the double reduction method is how to define the comprehensive safety factor based on two reduction parameters. The trajectory reduction method developed by Isakov can be used to ensure the minimum comprehensive safety factor on different conditions. However, its main shortcoming is that the method needs expensive calculation to determine the safety factor for a certain slope configuration. The paper examines the relationship between the comprehensive safety factor and cohesive and internal friction angle of soil, by using the FEM and trajectory method to calculate the minimum safety factor and corresponding reduction factor with respect to different inclinations of the slope. The initial strength effect on double reduction parameters are analyzed accordingly. The result shows for a certain slope configuration; the initial strength has little effect on the critical strength which is related to the minimum comprehensive safety factor. It means that for a slope with a certain inclination, even if the strength of soil is different, the critical strength is identical. The critical strength of soil slope is linear with the inclination of the slope, which means that every inclination corresponds to one critical cohesive and one critical internal friction angle. Consequently, a novel method to calculate the minimum safety factor is proposed in this paper. The result obtained by this method is close to the result which is from the limit equilibrium method, and compared with the original method by Isakov, this alternative method can simplify the calculation, and keep the result as accurate as the limit equilibrium method. Thus, it can be used to analyze the stability of slope.
- slope stability analysis /
- double reduction method /
- trajectory reduction method /
- safety factor /
- critical failure strength

HTML全文

图 1 黏土边坡强度折减路径

M_min或M_k分别代表最短路径法或同步折减法的强度折减路径与临界状态线的交点, k_C, k_φ分别代表黏聚力和内摩擦角正切值的折减系数, (1, 1)点代表未折减的初始状态, 蓝线表示同步折减方法的折减路径, 而红线最短路径往往并非沿着45°线同步折减

Figure 1. Trajectories of soil strength reduction in embankment composed of clay

下载: 全尺寸图片幻灯片

图 2 有限元强度折减法计算中的最优边界条件

H.模型高度；h.坡高；L₁，L₂.坡顶及坡底的长度

Figure 2. Optimal condition in FEM of strength reduction method

下载: 全尺寸图片幻灯片

图 3 内摩擦角正切值与折减系数的关系

Figure 3. Relationship between tangent of internal friction and reduction parameter

下载: 全尺寸图片幻灯片

图 4 黏聚力与折减系数的关系

Figure 4. Relationship between cohesive strength and reduction parameter

下载: 全尺寸图片幻灯片

图 5 坡度与临界内摩擦角正切值的关系

Figure 5. Relationship between slope inclination and tangent of critical internal frictionangle

下载: 全尺寸图片幻灯片

图 6 坡度与临界黏聚力的关系

Figure 6. Relationship between slope inclination and critical cohesive strength

下载: 全尺寸图片幻灯片

图 7 不同坡度下的强度折减系数与路径(红线表示最短折减路径, 蓝线表示同步折减路径)

Figure 7. Reduction parameters and trajectory with various slope inclination

下载: 全尺寸图片幻灯片

表 1 坡度与安全系数的关系

Table 1. Relationship between slope inclination and the safety factor

坡度/(°)	30	35	40	45	50	55	60	65	70
安全系数	2.422	2.214	2.051	1.921	1.802	1.701	1.606	1.522	1.355

下载: 导出CSV

表 2 不同黏性土在同一坡度下的最小安全系数对应的折减系数

Table 2. Reduction parameter of the minimum safety factor in the same slope inclination of various clay

不同黏土强度参数组合	tan20°		tan22°		tan24°		tan26°		tan28°
不同黏土强度参数组合	k_C	k_φ	k_C	k_φ	k_C	k_φ	k_C	k_φ	k_C	k_φ
C60	2.700	1.308	2.700	1.450	2.700	1.598	2.700	1.760	2.700	1.910
C55	2.475	1.308	2.475	1.450	2.475	1.598	2.475	1.760	2.475	1.910
C50	2.250	1.308	2.250	1.450	2.250	1.598	2.250	1.760	2.250	1.910
C45	2.025	1.308	2.025	1.450	2.025	1.598	2.025	1.760	2.025	1.910
C40	1.800	1.308	1.800	1.450	1.800	1.598	1.800	1.760	1.800	1.910
C35	1.575	1.308	1.575	1.450	1.575	1.598	1.575	1.760	1.575	1.910
C30	1.385	1.270	1.430	1.360	1.440	1.490	1.570	1.490	1.460	1.750
注：C和tanφ分别代表黏聚力和内摩擦角正切值, 如C60、tan20°分别表示初始黏聚力为60 kPa, 内摩擦角为20°的正切值

下载: 导出CSV

表 3 不同黏性土在同一坡度下最小安全系数对应临界破坏强度

Table 3. Critical strength of the minimum safety factor in the same slope inclination of various clay

不同黏土强度参数	tan20°		tan22°		tan24°		tan26°		tan28°
不同黏土强度参数	C_c	tanφ_c	C_c	tanφ_c	C_c	tanφ_c	C_c	tanφ_c	C_c	tanφ_c
C60	22.2	0.278	22.2	0.279	22.2	0.279	22.2	0.277	22.2	0.278
C55	22.2	0.278	22.2	0.279	22.2	0.279	22.2	0.277	22.2	0.278
C50	22.2	0.278	22.2	0.279	22.2	0.279	22.2	0.277	22.2	0.278
C45	22.2	0.278	22.2	0.279	22.2	0.279	22.2	0.277	22.2	0.278
C40	22.2	0.278	22.2	0.279	22.2	0.279	22.2	0.277	22.2	0.278
C35	22.2	0.278	22.2	0.279	22.2	0.279	22.2	0.277	22.2	0.278
C30	21.7	0.287	21.0	0.297	20.8	0.299	19.1	0.327	20.5	0.304
注：C_c、tanφ_c分别为临界黏聚力(kPa)和内摩擦角正切值，而C60、tan20°分别表示初始黏聚力为60 kPa, 内摩擦角为20°的正切值

下载: 导出CSV

表 4 不同坡度下的临界强度值

Table 4. Critical strength in the various slope inclination

坡度/(°)	C_c/kPa	tanφ_c
50	24.038	0.298
45	22.222	0.278
40	20.513	0.259
35	19.231	0.234
30	18.018	0.206
25	18.433	0.163

下载: 导出CSV

表 5 不同方法计算的边坡安全系数结果对比

Table 5. Comparison of calculation results with different methods

坡度	35°	40°	45°
极限平衡法	1.70	1.61	1.50
最短折减路径法	1.652	1.525	1.436
本研究改进后的方法	1.668	1.538	1.422

下载: 导出CSV

参考文献(24)

[1]	Zienkiewicz O C, Chumpheson O, Clewis R W. Associated and non-associated visco-plasticity and plasticity in soil mechanics[J]. Geotechnique, 1975, 25(4): 671-689. doi: 10.1680/geot.1975.25.4.671
[2]	Griffiths D V, Lane P A. Slope stability analysis by finite elements[J]. Geotechnique, 1999, 49(3): 387-403. doi: 10.1680/geot.1999.49.3.387
[3]	郑宏, 田斌, 刘德富等. 关于有限元边坡稳定性分析中安全系数的定义问题[J]. 岩石力学与工程学报, 2005, 24(13): 2225-2230. doi: 10.3321/j.issn:1000-6915.2005.13.004 Zheng H, Tian B, Liu D F, et al. On definitions of safety factor of slope stability analysis with finite element method[J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(13): 2225-2230(in Chinese with English abstract). doi: 10.3321/j.issn:1000-6915.2005.13.004
[4]	郑宏, 李春光, 李焯芬, 等. 求解安全系数的有限元法[J]. 岩土工程学报, 2002, 24(5): 626-628. doi: 10.3321/j.issn:1000-4548.2002.05.020 Zheng H, Li C G, Li C F, et al. Finite element method for solving the factor of safety[J]. Chinese Journal of Geotechnical Engineering, 2002, 24(5): 626-628(in Chinese with English abstract). doi: 10.3321/j.issn:1000-4548.2002.05.020
[5]	郑宏, 葛修润, 谷先荣, 等. 关于岩土工程有限元分析中的若干问题[J]. 岩土力学, 1995, 16(3): 7-11. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX503.001.htm Zheng H, Ge X R, Gu X R, et al. Some problems in FEA for geotechnical engineering[J]. Rock and Soil Mechanics, 1995, 16(3): 7-11(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX503.001.htm
[6]	赵尚毅, 郑颖人, 时卫民, 等. 用有限元强度折减法求边坡稳定安全系数[J]. 岩土工程学报, 2002, 24(3): 343-346. doi: 10.3321/j.issn:1000-4548.2002.03.017 Zhao S Y, Zheng Y R, Shi W M, et al. Slope safety factor analysis by strength reduction FEM[J]. Chinese Journal of Geotechnical Engineering, 2002, 24(3): 343-346(in Chinese with English abstract). doi: 10.3321/j.issn:1000-4548.2002.03.017
[7]	连镇营, 韩国城, 孔宪京. 强度折减有限元法研究开挖边坡的稳定性[J]. 岩土工程学报, 2001, 23(4): 406-411. https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC200104004.htm Lian Z Y, Han G C, Kong X J. Stability analysis of excavation by strength reduction FEM[J]. Chinese Journal of Geotechnical Engineering, 2001, 23(4): 406-411(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-YTGC200104004.htm
[8]	郑颖人, 赵尚毅, 宋雅坤. 有限元强度折减法研究进展[J]. 后勤工程学院学报, 2005(3): 1-6. doi: 10.3969/j.issn.1672-7843.2005.03.001 Zheng Y R, Zhao S Y, Song Y K. Advance of study on the strength reduction finite element method[J]. Journal of Logistical Engineering University, 2005(3): 1-6(in Chinese with English abstract). doi: 10.3969/j.issn.1672-7843.2005.03.001
[9]	赵尚毅. 有限元强度折减法及其在土坡与岩坡中的应用[D]. 重庆: 后勤工程学院, 2005. Zhao S Y. Strength reduction finite element method and its application to soil and rock slopes[D]. Chongqing: Logistical Engineering University of PLA, 2005(in Chinese with English abstract).
[10]	张鲁渝, 郑颖人, 赵尚毅, 等. 有限元强度折减系数法计算土坡稳定安全系数的精度研究[J]. 水利学报, 2003(1): 21-27. doi: 10.3321/j.issn:0559-9350.2003.01.005 Zhang L Y, Zheng Y R, Zhao S Y, et al. The feasibility study of strength reduction method with FEM for calculating safety factors of soil slope stability[J]. Journal of Hydraulic Engineering, 2003(1): 21-27(in Chinese with English abstract). doi: 10.3321/j.issn:0559-9350.2003.01.005
[11]	蒋先平, 张鹏, 卢艺伟, 等. 物质点强度折减法边坡失稳判据选择方法[J]. 地质科技通报, 2022, 41(2): 113-122. doi: 10.19509/j.cnki.dzkq.2021.0075 Jiang X P, Zhang P, Lu Y W, et al. Slope failure criterion for the strength reduction material point method[J]. Bulletin of Geological Science and Technology, 2022, 41(2): 113-122(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2021.0075
[12]	Yuan W, Bai B, Li X C, et al. A strength reduction method based on double reduction parameters and its application[J]. J. Cent. South. Univ. Technol., 2013, 20(9): 2555-2562. doi: 10.1007/s11771-013-1768-4
[13]	Bai B, Yuan W, Li X C. A new double reduction method for slope stability analysis[J]. J. Cent. South. Univ. Technol., 2014, 21: 1158-1164. doi: 10.1007/s11771-014-2049-6
[14]	白冰, 袁维, 石露, 等. 一种双折减法与经典强度折减法的关系[J]. 岩土力学, 2015, 36(5): 1275-1281. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201505006.htm Bai B, Yuan W, Shi L, et al. Comparing a new double reduction method to classic strength reduction method for slope stability analysis[J]. Rock and Soil Mechanics, 2015, 36(5): 1275-1281(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201505006.htm
[15]	Taylor D W. Fundamentals of soil mechanics[M]. NewYork: John Wiley and Sons, 1948.
[16]	Isakov A, Moryachkov Y. Estimation of slope stability using two-parameter criterion of stability[J]. International Journal of Geomechanics, 2014, 14(3): 613-624.
[17]	郑颖人, 赵尚毅. 边(滑)坡工程设计中安全系数的讨论[J]. 岩石力学与工程学报, 2006, 25(9): 1937-1940. doi: 10.3321/j.issn:1000-6915.2006.09.032 Zheng Y R, Zhao S Y. Discussion on safety factors of slope and landslide engineering design[J]. Chinese Journal of Rock Mechanics and Engineering, 2006, 25(9): 1937-1940(in Chinese with English abstract). doi: 10.3321/j.issn:1000-6915.2006.09.032
[18]	唐芬, 郑颖人. 边坡渐进破坏双折减系数法的机理分析[J]. 地下空间与工程学报, 2008, 4(3): 436-441. doi: 10.3969/j.issn.1673-0836.2008.03.009 Tang F, Zheng Y R. Mechanism analysis on dual reduction factors about the progressive failure of slope[J]. Chinese Journal of Underground Space and Engineering, 2008, 4(3): 436-441(in Chinese with English abstract). doi: 10.3969/j.issn.1673-0836.2008.03.009
[19]	唐芬, 郑颖人, 赵尚毅. 土坡渐进破坏的双安全系数讨论[J]. 岩土工程学报, 2007, 26(7): 1402-1407. https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200707013.htm Tang F, Zheng Y R, Zhao S Y. Discussion on two safety factors for progressive failure of soil slope[J]. Chinese Journal of Rock Mechanics and Engineering, 2007, 26(7): 1402-1407(in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-YSLX200707013.htm
[20]	Usluogullari O F. A novel algorithm for slope stability analysis[J]. Proceedings of the Institution of Civil Engineers Ground Improvement, 2016, 169(1): 3-14.
[21]	赵炼恒, 曹景源. 唐高朋, 等. 基于双强度折减策略的边坡稳定性分析方法探讨[J]. 岩土力学, 2014, 35(10): 2977-2984. https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201410031.htm Zhao L H, Cao J Y, Tang G P, et al. Discussion on slope stability analysis with double strength reduction technique[J]. Rock and Soil Mechanics, 2014, 35(10): 2977-2984(in Chinese with English abstract) https://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201410031.htm
[22]	Fang H, Chen Y F, Xu G, et al. New instability criterion for stability analysis of homogeneous slopes with double strength reduction[J]. International Journal of Geomechanics, 2020, 20(9): 04020162.
[23]	Meng Q, Wang H, Cai M, et al. Multiscale strength reduction method for heterogeneous slope using hierarchical FEM/DEM modeling[J]. Computers and Geotechnics, 2019, 115: 103164.
[24]	谭明健, 周春梅, 孙东, 等. 软硬互层顺层岩质边坡破坏试验[J]. 地质科技通报, 2022, 41(2): 274-281, 324. doi: 10.19509/j.cnki.dzkq.2021.0096 Tan M, Zhou C, Sun D, et al. Failure experiment of soft- hard interlayer bedding rock slope[J]. Bulletin of Geological Science and Technology, 2022, 41(2): 274-281, 324(in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2021.0096