水平定向钻隧道地质勘察孔岩屑运移与地层相关性研究

王震; 崔景川; 彭文波; 闫雪峰; 马保松; 李信杰

doi:10.19509/j.cnki.dzkq.2022.0114

水平定向钻隧道地质勘察孔岩屑运移与地层相关性研究

doi: 10.19509/j.cnki.dzkq.2022.0114

1.
中国交通建设股份有限公司, 北京 100088
2.
中交第二公路勘察设计研究院有限公司, 武汉 430056
3.
中国地质大学(武汉)工程学院, 武汉 430074
4.
中山大学土木工程学院, 广东珠海 510275

基金项目:

国家自然科学基金青年基金项目 42002284

新疆维吾尔自治区重大科技专项 2020A03003-1

详细信息

作者简介:
王震(1982-), 男, 高级工程师, 主要从事工程项目管理工作。E-mail: 15871744068@139.com

通讯作者:
闫雪峰(1989-), 男, 讲师, 主要从事非开挖工程理论与技术相关研究工作。E-mail: cugyanxf@163.com

中图分类号: P634.5
计量
- 文章访问数: 855
- PDF下载量: 59
- 被引次数: 0
出版历程
- 收稿日期: 2021-06-29

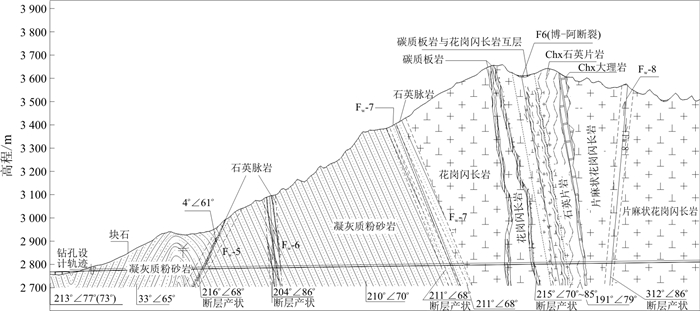
Correlation study of cuttings and formation during tunnel geological investigation using HDD technology

1.
China Communications Construction Company Limited, Beijing 100088, China
2.
CCCC Second Highway Consultants Co., Ltd, Wuhan 430056, China
3.
Faculty of Engineering, China University of Geosciences(Wuhan), Wuhan 430074, China
4.
School of Civil Engineering, Sun Yat-Sen University, Zhuhai Guangdong 510275, China

摘要

摘要:
为准确反演勘察孔地层岩性信息、提高岩屑反演勘察孔地层岩性的准确性, 依托某隧道水平定向钻地质勘察项目, 开展了水平定向钻隧道地质勘察孔岩屑运移与地层相关性研究。研究结果表明: 依据岩屑运移速度、费祥俊临界流速理论和Larsen最小返速理论对现场岩屑运移形式的分析, 推知不同围岩岩屑颗粒在水平定向钻孔环空内的运移形式以推移为主, 少部分悬移, 且存在少量岩屑床; 在水平定向钻勘察工程现场, 钻头处产生的地层岩屑最先以悬浮运移状态随泥浆返出; 基于物料输送相关理论分析岩屑颗粒自由悬浮和运动方程, 建立了水平定向钻勘察孔岩屑悬移运移模型; 依托现场碳质板岩和石英片岩相关参数进行了岩屑运移与地层相关性分析, 分别得出完整地层和断层破碎带的岩屑运移孔壁阻力系数, 且断裂带处岩屑运移孔壁阻力系数约为非断裂带处的2.72倍; 得出不同直径钻杆工作范围内的钻孔环空岩屑最终悬浮运移速度, 建立了岩屑运移与地层的关联分析计算模型。研究结果为准确反演勘察孔地层岩性信息, 以及后续隧道的顺利施工提供了理论和数据支持。
- 水平定向钻 /
- 隧道地质勘察 /
- 岩屑运移 /
- 地层反演
Abstract:
To improve the accuracy of formation inversion by cuttings during tunnel geological investigation using horizontal directional drilling technology, this study focuses on the correlation of cutting transportation features and formation spatial locations based on a tunnel geological investigation project. This study mainly draws the following conclusions: The different cuttings' migration modes are determined according to the cutting's migration settling velocity, Fei Xiangjun's critical velocity theory, and Larsen's minimum return velocity theory. The migration of cuttings in the annulus is bedload, a small number of cuttings are suspended load, and the little remaining is cutting's bed. The newly generated cuttings at the drill bit tooface would come out as suspended load first with drilling fluid in the field. The cutting suspended moving mode was established based on the material transportation theory, the freesuspension, and the movement equations of cutting particles. According to the recorded data of carbonaceous slate cuttings and quartz schist cuttings, the resistance coefficient of the intact borehole wall during cutting movement is obtained, while the resistance coefficient of the fault zone is 2.72 times that of the intact zone. Then, the final suspended moving speeds of cuttings in the annulus for Φ140 mm and Φ168 mm drill rods are calculated, and finally, the correlation analysis calculation model for cuttings and formation is established.
- horizontal directional drilling /
- tunnel geological investigation /
- cuttings transportation /
- formation inversion

HTML全文

图 1 水平定向钻勘察工程剖面图

Figure 1. Sectional view of the horizontal directional drilling survey project

下载: 全尺寸图片幻灯片

图 2 钻孔环空岩屑颗粒受力示意图

u_w.泥浆流速; m.颗粒质量; W′.有效重力; F_D.水流拖拽力；F_L.流体上举力

Figure 2. Schematic diagram of the force on the cutting particles in the borehole annulus

下载: 全尺寸图片幻灯片

图 3 水平钻孔内单颗粒受力与加速示意图

t为时间；L为颗粒在水平钻孔环空内平行流中的运动距离；其余量符号说明同图 2

Figure 3. Schematic diagram of the force and acceleration of a single particle in a horizontal borehole

下载: 全尺寸图片幻灯片

图 4 水平定向钻孔内颗粒群受力及运动示意图(ΔL.微段长度)

Figure 4. Schematic diagram of the force and movement of the particle group in the horizontal directional borehole

下载: 全尺寸图片幻灯片

图 5 碳质板岩钻孔(Φ140钻杆)环空岩屑颗粒加速段时间与速度关系曲线

Figure 5. Relationship curve between acceleration time and velocity of cutting particles in the annulus of carbonaceous slate drilling(Φ140 drill pipe)

下载: 全尺寸图片幻灯片

图 6 碳质板岩钻孔(Φ140钻杆)环空岩屑颗粒加速段距离与速度关系曲线

Figure 6. Relationship curve between the distance and velocity of the acceleration section of the cutting particles in the annulus of the carbonaceous slate bore(Φ140 drill pipe)

下载: 全尺寸图片幻灯片

表 1 现场工况参数

Table 1. List of on-site working condition parameters

岩屑岩性	岩屑容重/(kN·m^-3)	泥浆马氏漏斗黏度/s	泥浆动力黏度/(Pa·s)	泥浆相对密度	泵量/(m³·h^-1)	钻速/(m·h^-1)	初次出现钻孔深度/m
碳质板岩	26.7	47	0.006	1.02	108	11.27	1 748.26
石英片岩	28.1	32	0.003	1.01	108	8.84	2 026.09

下载: 导出CSV

表 2 岩屑统计运移时间

Table 2. Statistical migration time of cuttings

岩屑岩性	长度/m	运移时间/min	平均运移速度/(m·s^-1)	Ф140钻杆环空泥浆流速/(m·s^-1)
碳质板岩	1 748.26	42	0.69	0.89
石英片岩	2 026.09	55	0.61	0.89

下载: 导出CSV

表 3 单颗粒自由悬浮速度的不规则修正系数^[19]

Table 3. Irregular correction coefficients of the free suspension velocity of single particles

序号	物体名称	形状修正系数K
1	半球形	1.76
2	圆柱体	1.52
3	棱形体	1.76
4	正方形体	1.86
5	正方形板片	3.30
6	不规则球体	1.20
7	不规则椭圆体	1.10

下载: 导出CSV

表 4 实验指数β与颗粒雷诺数Re_p的关系^[19]

Table 4. Relationship between the experimental index β and particle Reynolds number Re_p

u₀公式	斯托克斯公式			阿连公式			牛顿公式
Re_p	0.01	0.1	1	10	100	1 000	10⁴	10⁵
β	4.6	4.5	4.2	3.6	3.1	2.5	2.3	2.3

下载: 导出CSV

表 5 基于现场岩屑运移的地层反演分析计算

Table 5. Analysis and calculation of the time-effect of on-site cutting migration

岩屑岩性	长度/m	Ф140钻杆环空长度/m	Ф168钻杆环空长度/m	统计运移时间t/min	里程分段/m	Ф140钻杆环空		Ф168钻杆环空		孔壁阻力系数λ_s
岩屑岩性	长度/m	Ф140钻杆环空长度/m	Ф168钻杆环空长度/m	统计运移时间t/min	里程分段/m	固液速度比	最终悬浮运移速度/(m·s^-1)	固液速度比	最终悬浮运移速度/(m·s^-1)	孔壁阻力系数λ_s
碳质板岩	1 748.26	668.5	1 079.76	42	[0, 1 079.76) [1 079.76, 1 748.26]	/ 0.69	/ 0.61	0.68 /	0.76 /	1 211 1 211
石英片岩	2 026.09	668.5	1 357.59	55	[0, 1 357.59) [1 357.59, 1 748.26) [1 748.26, 2 026.09]	/ 0.63 0.50	/ 0.56 0.44	0.61 / /	0.69 / /	1 211 1 211 3 295

下载: 导出CSV

参考文献(19)

[1]	马保松, 闫雪峰, 曾聪, 等. 一种采用水平定向钻的工程地质勘察方法[P]. 湖北省: CN110578468B, 2021-03-26. Ma B S, Yan X F, Zeng C, et al. An engineering geological survey method using horizontal directional drilling[P]. Hubei Province: CN110578468B, 2021-03-26(in Chinese).
[2]	马保松, 程勇, 刘继国, 等. 超长距离水平定向钻进技术在隧道精准地质勘察的研究及应用[J/OL]. 隧道建设(中英文): 1-10[2021-03-19](2021-06-29). http://kns.cnki.net/kcms/detail/44.1745.U.20210311.1322.002.html. Ma Baosong, Cheng Yong, Liu Jiguo, et al. Research and Application of Tunnel Accurate Geological Investigation Using HDD Technology[J/OL]. Tunnel Construction (Chinese and English): 1-10[2021-03-19](2021-06-29). http://kns.cnki.net/kcms/detail/44.1745.U.20210311.1322.002.html(in Chinese with English abstract).
[3]	康坤坤, 钱锋, 尤伟星, 等. 水平定向钻孔壁稳定性影响因素分析[J]. 地质科技情报, 2016, 35(2): 45-48. https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201602011.htm Kang K K, Qian F, You W X, et al. Influencing factors for the horizontal directional drilling hole wall stability[J]. Geological Science and Technology Information, 2016, 35(2): 45-48 (in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-DZKQ201602011.htm
[4]	颜慧明, 常威, 郭绪磊, 等. 岩溶水流系统识别方法及其在引调水工程隧洞选线中的应用[J]. 地质科技通报, 2022, 41(1): 127-136. doi: 10.19509/j.cnki.dzkq.2022.0008 Yan H M, Chang W, Guo X L, et al. Identification of the karst water system and its application in the tunnel line selection of water diversion projects[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 127-136 (in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0008
[5]	Ford J T, Peden J M, Oyeneyin M B, et al. Experimental investigation of drilled cuttings transport in inclined boreholes[C]//Anon. SPE annual technical conference and exhibition. [S. l.]: Society of Petroleum Engineers, 1990.
[6]	Ibrahim A A, Musa T A. Experimental study optimizing hole cleaning-cuttings transport in oil drilling engineering[J]. Journal of China University of Geosciences, 2003, 14(2): 182-189.
[7]	Yu M, Takach N E, Nakamura D R, et al. An experimental study of hole cleaning under simulated downhole conditions[C]//Anon. SPE annual technical conference and exhibition. [S. l.]: Society of Petroleum Engineers, 2007.
[8]	张好林, 李根生, 肖莉, 等. 水平井中钻柱旋转对岩屑运移影响规律研究[J]. 科学技术与工程, 2016, 16(2): 125-130. https://www.cnki.com.cn/Article/CJFDTOTAL-KXJS201602025.htm Zhang H L, Li G S, Xiao L, et al. Study on the influence of the rotation of drill string on cuttings transportation in horizontal well[J]. Science Technology and Engineering, 2016, 16(2): 125-130 (in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-KXJS201602025.htm
[9]	汤捷. 水平井岩屑颗粒启动及运移规律研究[D]. 黑龙江大庆: 东北石油大学, 2019. Tang J. Study on initiation and migration of particles on cuttings bed in horizontal wells[D]. Daqing, Heilongjiang: Northeast Petroleum University, 2019 (in Chinese with English abstract).
[10]	刘玉明, 管志川, 呼怀刚. 大位移井岩屑运移研究综述与展望[J]. 科学技术与工程, 2015, 15(28): 88-95, 102. doi: 10.3969/j.issn.1671-1815.2015.28.016 Liu Y M, Guan Z C, Hu H G. Review of hole cleaning research in extended-reach drilling[J]. Science Technology and Engineering, 2015, 15(28): 88-95, 102 (in Chinese with English abstract). doi: 10.3969/j.issn.1671-1815.2015.28.016
[11]	Gavignet A A, Sobey I J. Model aids cuttings transport prediction[J]. Journal of Petroleum Technology, 1989, 41(9): 916-921. doi: 10.2118/15417-PA
[12]	Martins A L, Santana C C. Evaluation of cuttings transport in horizontal and near horizontal wells: A dimensionless approach[C]//Anon. SPE Latin America petroleum engineering conference. [S. l.]: Society of Petroleum Engineers, 1992.
[13]	Nguyen D, Rahman S S. A three-layer hydraulic program for effective cuttings transport and hole cleaning in highly deviated and horizontal wells[C]//Anon. SPE/IADC Asia Pacific Drilling Technology. [S. l.]: Society of Petroleum Engineers, 1996.
[14]	汪志明, 张政. 水平井两层稳定岩屑传输规律研究[J]. 石油大学学报: 自然科学版, 2004, 28(4): 63-66. https://www.cnki.com.cn/Article/CJFDTOTAL-SYDX200404015.htm Wang Z M, Zhang Z. A model for two-layer cutting transport in horizontal wells[J]. Journal of the University of Petroleum: Edition of Natural Science, 2004, 28(4): 63-66 (in Chinese with English abstract). https://www.cnki.com.cn/Article/CJFDTOTAL-SYDX200404015.htm
[15]	倪晓东. 水平井段岩屑瞬态运移规律研究[D]. 黑龙江大庆: 东北石油大学, 2017. Ni X D. Study on transient migration law of cuttings in horizontal well section[D]. Daqing, Heilongjiang: Northeast Petroleum University, 2017 (in Chinese with English abstract).
[16]	曾斌, 陈植华, 邵长杰, 等. 基于地下水流系统理论的岩溶隧道涌突水来源及路径分析[J]. 地质科技通报, 2022, 41(1): 99-108. doi: 10.19509/j.cnki.dzkq.2022.0006 Zeng B, Chen Z H, Shao C J, et al. Analysis of source and path of water inrush in karst tunnel based on the theory of groundwater flow system[J]. Bulletin of Geological Science and Technology, 2022, 41(1): 99-108 (in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2022.0006
[17]	黄建华, 鲍锋, 王蕴晨, 等. 并行差异断面顶管施工对地表变形的影响[J]. 地质科技通报, 2021, 40(6): 185-192. doi: 10.19509/j.cnki.dzkq.2021.0618 Huang J H, Bao F, Wang W C, et al. Influence of ground-surface deformation in pipe jacking construction with parallel differential section[J]. Bulletin of Geological Science and Technology, 2021, 40(6): 185-192 (in Chinese with English abstract). doi: 10.19509/j.cnki.dzkq.2021.0618
[18]	费祥俊. 浆体与粒状物料输送水力学[M]. 北京: 清华大学出版社, 1994. Fei X J. Hydraulics of slurry and granular material transportation[M]. Beijing: Tsinghua University Press, 1994(in Chinese).
[19]	Larsen T I, Pilehvari A A, Azar J J. Development of a new cuttings-transport model for high-angle wellbores including horizontal wells[J]. SPE Drilling & Completion, 1997, 12(2): 129-136.